Mortar shell dynamics
by Walter J. Eldredge
[Walter Eldredge is the son of the late Paul J. Eldredge, who served in Co D of the 2nd Cml Mortar Bn in Europe during WWII. This article was first published in the February 2003 issue of The Red Dragon, the newsletter of the 2nd Chemical Mortar Battalion Association.]
Over dinner at the last reunion the question came up “Why does a 4.2 shell turn downward in flight and impact nose first?” As it happened my oldest son and I had spent a long time discussing that question and thought we had the answer. I sent a long technical analysis to Bruce Elliott who asked me if I could boil it down for this issue of The Red Dragon. So here goes.
A spinning shell has to play by some well-defined rules called “free body dynamics”. Rule 1 is that the spin will keep the shell at the same angle it had when it left the muzzle, unless there is some force to change that angle. (That's why kid's spinning gyroscopes and tops will stand up instead of falling over.) Rule 2 is that when a force like that is applied to the shell, the shell's center of gravity keeps moving along the same trajectory, while the body of the shell wobbles or tumbles around it.
The force required by Rule 1 comes from the air. In flight the shell feels a headwind of something like 600 miles an hour, which generates one heck of a force – stick your hand out the window of a car doing 60 and multiply that times 100 (not 10; 100 – force goes as the square of windspeed). We all know that one effect of that force is to slow the shell down. The other effect is that, unless the shell is pointed directly along the trajectory, then the wind is “off center” from the shell's point of view, and it exerts a sideways, or rotating, force on the shell.
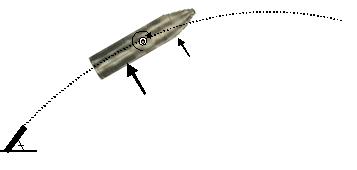That force makes the shell rotate about its center of gravity. The CG, as it's called, is the balance point of the shell. If you drilled a series of holes from side to side through the shell and stuck a steel rod through each one in turn, you would find one where the shell would hang perfectly level because there is the same amount weight in front of the rod as behind it – that's the CG. It's represented by the white circle in the figure.
Now when a shell is fired and first clears the muzzle, the “headwind” is right on the nose, and there is no force to change the shell's angle. But the shell doesn't continue to travel in a straight line from the muzzle – it follows a curved trajectory, because it's trying to fly up and forward while gravity is pulling it down and the air drag is pulling it backward. As soon as that trajectory starts to curve, the wind is no longer dead on the shell's nose. It's a little off center and now we have the force required by Rule 1.
According to Rule 2, this will make the shell rotate around the CG. Which way will it rotate? Although there is equal weight fore and aft of the CG, there is more cross-sectional area behind the CG. So that sideways wind pushes the rear of the shell a little harder than the nose, and rotates the shell back in line with the headwind. Once in line, there's no more force and the shell keeps that orientation – until there is a little more curve, and the same thing happens again. And again, with the shell's angle being adjusted constantly to keep it pointing along the line of the trajectory.
Another way to think of this is to remember that shell with the rod stuck through the hole at the CG. It hangs level in still air, but now suppose you stand in front of the shell with an electric fan blowing 600 miles per hour. As you move the fan up, so it's blowing down on the shell, the nose of the shell is going to turn up into the wind. Move the fan down, and the nose of the shell will turn downwards. It will always point directly into that headwind.
Let's play with this idea a little bit. What if you fired a mortar on the moon? There would be no air, so no force generated. Gravity would pull the shell downward through a trajectory (although more like the trajectory of a 15-mm rifle than a mortar). But under rule 1 the shell will fly, and land, with its nose pointed upward at exactly the same angle it had when it cleared the muzzle. Have you ever seen a spinning football fly that way, coming down at the same angle it had initially? That's because the CG of the football is dead center, and the cross sectional area in front of it is equal to the cross-sectional area behind it. When the trajectory causes the “wind” to be off center, the force on the back half pushing it into line with the trajectory is the same as the force on the front half in the other direction. Plus, of course, the ball isn't traveling at 600 miles per hour so the wind force is a lot smaller.
What if you made a shell out of balsa wood with a lead plate on the bottom, so that the CG was way in the back and there was more cross-sectional area in front of it than behind it? The rotating force on that shell would cause it to turn around and fly tail first.
And lastly, what about smooth bores? The Stokes mortars of World War I fired a cylindrical shell. Any little looseness or imperfection and the shell left the barrel with a tumbling motion. With no spin, Rule 1 didn't apply and they just kept on tumbling until they hit. In fact they had an “all-ways” impact fuze that would detonate at any orientation. Later the 60-mm and 81-mm mortar shells were given “stabilizing fins” that acted like little rudders to resist that tumbling. As a side effect they provided a force that, under rule 1, kept the shell oriented along the trajectory. They also reduced the payload, since the shell had to taper at the rear to accommodate the fins. The British 4.2 mortar of World War II and Korea was smooth bore, and its tapered shell with fins carried about one third of the WP or HE payload of the American 4.2.